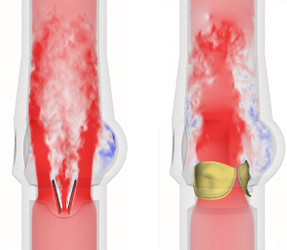
Mike Lee’s paper, On the Lagrangian-Eulerian coupling in the immersed finite element/difference method, has been accepted to appear in Journal of Computational Physics. (A preprint is available on the arXiv.) This paper systematically tests the accuracy of the immersed finite element/difference method (IBFEMethod in IBAMR) using different regularized delta functions and relative mesh densities for several benchmark problems along with our large-scale model of a bioprosthetic heart valve in a pulse duplicator. Our results indicate that kernels satisfying a commonly imposed even–odd condition require higher resolution to achieve similar accuracy as kernels that do not satisfy this condition. We also find that narrower kernels are more robust, in the sense that they yield results that are less sensitive to relative changes in the Eulerian and Lagrangian mesh spacings, and that structural meshes that are substantially coarser than the Cartesian grid can yield high accuracy for shear-dominated cases but not for cases with large normal forces. I think this will be a very useful study for practitioners of the IB method.
Congratulations, Mike!